This article was originally published on Domus 1058, June 2021.

Mathematicians and architects have traditionally used two distinct methods for the study and manipulation of geometric form. Analytic geometry, also known as coordinate geometry, describes and modulates form through algebraic equations. Descriptive geometry (related to the subject known since antiquity as solid geometry) operates via more direct graphic means, constructing projective representations directly with the drawing tools of compass and straight edge. Understanding the historical and contemporary reciprocity between these methods is important for architects.

As digital modelling and computation seem to liberate design processes by facilitating the production of more complex and analytical forms, axioms of geometry continue to constrain outcomes due to intractable issues of representation, materiality and construction. René Descartes (1596-1650) is generally credited with the invention of analytic geometry, yet the subject’s antecedents date back to ancient Greece – most notably when Menaechmus (380-320 BCE) devised an elegant solution to the Delian problem involving the construction and intersection of two curves, a parabola and a hyperbola – both conic sections. As the geometric embodiment of the solution space of algebraic expressions, conics were critical to the development of analytic geometry, describing many behaviours of Newtonian mechanics. From celestial orbits (elliptical) to gravitational acceleration, projectiles, optics and acoustics (parabolic), to GPS tracking, heliostats (hyperbolic), the breadth of their usefulness cannot be overstated.

Two millennia later, Descartes’s concise mathematical text La Géométrie (1637) provided methods to fuse algebra and geometry through the crucial idea of a coordinate system, allowing polynomial expressions to be graphed in 2D and 3D. The authoring of analytic geometry occurs in algebraic notation, yet the visualisation of its function curves and surfaces has always been an integral part of the discipline. All equations could now be graphically represented by points, curves or surfaces. Conversely, all related geometric points, curves and surfaces could be mathematically described via algebraic notation.

When contemplating such deterministic relationships between written notation and geometric form, an architect might consider various parallels in contemporary practice such as the reciprocity between a BIM spreadsheet and an architectural proposal, or between a Grasshopper script and a parameterised surface. While analytic geometry employs both algebraic notation and visual representation, descriptive geometry is ensconced in the latter. As a radical restatement of solid geometry (Robin Evans, The Projective Cast), the publication of Géométrie descriptive (1799) by Gaspard Monge (1746-1818) codified elegant techniques that allowed the architect and engineer to parse projective information economically, enabling a command of all dimensions of Euclidean space on the flat drawing surface. 

Descriptive geometry became the preferred method of design inquiry for the architect, who was empowered to explore an expanded range of challenging architectural and geometric ideas, yet it also served to further the estrangement between architecture and mathematics after the 18th-century rise of differential calculus. Cones and their section curves also played a major role in the development and dissemination of descriptive techniques. While vaulted and turret forms had been present in architectural construction since antiquity, ruled surfaces (a surface where at least one curve field U or V is composed of straight lines) such as the (singly ruled) cone and cylinder were of intensely renewed interest to the descriptive geometer. The presence of straight ruling lines on their surfaces meant that one need only manage the projections of two discrete points and one knew the location of the infinite points along that line.

Projective studies of ruled surfaces similar to those found in Monge’s text – cones, cylinders, conoids, helicoids, etc. – appeared in the many architectural treatises over 100 years before Géométrie descriptive. The same geometric property that enabled expedient projection in drawing was also instrumental for stereotomic construction (stone-cutting), which became more accomplished and structurally daring with the augmentation of descriptive techniques. The exposed outer surfaces of stone masonry construction were often curved in one axis and flat in the other. The presence of ruling lines in the non-curved axis allowed masons to precisely cut and dimensionally verify the fidelity surface with straight-edge tools. 

Thus, the tendency of descriptive representation to favour the use of ruled surfaces was reinforced by the pragmatic necessities of stereotomy. Digital computation and visualisation methods have been arguably more liberating for the contemporary architect than Monge’s methods were in the 18th century. The power and accessibility of digital design processes has enabled the architect to author complex form expediently and iteratively, seeming to finally eradicate the cumbersome constraints of Mongean projection and stereotomic (volumetric) construction which conspired to insist that we draw and build with at least some straight ruling lines. Yet architecture’s contemporary shift towards fabrication methods based on sheet material (not stone) for surface rendition has engendered new constrained and reciprocal relationships between design geometry and material construction. Unless there is material deformation, the accurate construction of contemporary curved surfaces depends largely on the geometric and material behaviour of isometric developability.

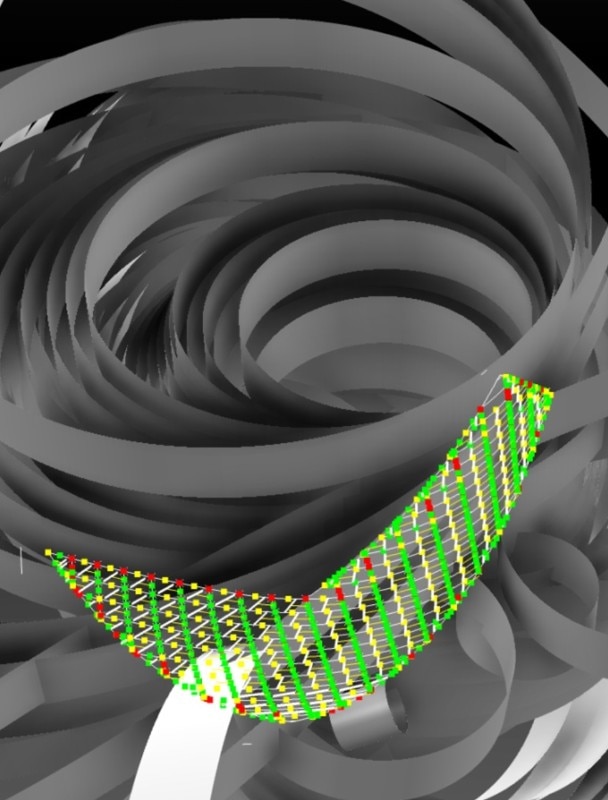
Most surfaces that have complex curvature during design phases must be post-rationalised into piece-wise patches of cones, cylinders or other developable ruled surfaces to amenably accept methods of contemporary construction. These processes are well known from practices such as Gehry Partners, and serve to exemplify the continued relevance of conical and ruled surfaces, even amid the digital computational prowess of contemporary practice. The summary conclusion after recounting this accelerated and disjointed history is that geometry cannot be fooled or bypassed, as its ubiquity and coherence is always present. The disciplines of analytic and descriptive geometry are two parts of an axiomatic system capable of describing infinite spatial methods and outcomes for the architect – from the Newtonian mechanics of the natural world to the abstraction of Mongean projection, to the material intelligence of construction. The stubborn and varied persistence of conical and ruled surfaces manifest throughout the history of architectural thought and practice is but one example of how irrefutable and widely applicable the logic of mathematics can be. In this sense, geometry demonstrates that its power to constrain is comparable to its power to liberate.

Cameron Wu Born in 1972 in Ohio, USA, Cameron Wu got a degree in civil engineering at the Princeton University School of Architecture (where he teaches design studios, courses in architectural geometry and representation, and formal analysis) and a master in architecture at the Harvard University. His independent architectural practice engages with typological and formal analysis of historical precedents and on-going research and design with developable and ruled surface geometries.

Opening Image: Gehry Partners, 3D simulations for the Fondation Louis Vuitton in Paris, 2014. Courtesy Gehry Technologies/Trimble Consulting
Preview image: A plate from La théorie et la pratique de la coupe des pierres by Amédée-François Frézier, 1754-1769. Courtesy © Bibliothèque nationale de France